|
The magic of perfect fifths
This lesson will help you learn how to identify and notate perfect fifths instantaneously, at sight without counting whole and half steps. This will help you speed up your analysis and notation of intervals and chords, and may improve your accuracy. All perfect intervals have these special qualities. In this lesson, I will focus on fifths, but in fact this same technique can be used with fourths as well. Here is the principle; we will discuss why it works below.
· Here is the special property: all fifths are perfect as long as the same accidentals are on both notes, (the accidentals “match”) unless the fifth consists of the notes are B & F.
This means that you can look at any fifth, and tell if it is perfect or not at sight, with no measuring of whole or half steps required.
· For all fifths (except B & F), if there are no sharps or flats on either note, it’s a perfect fifth. If both notes are sharped, such as the fifth G# and D#, the fifth is still perfect. Or if both notes are flatted, such as E-flat and B-flat, the fifth is also perfect. This matching principle works the same way even if both notes are double-sharped or double-flatted: the fifth is still perfect. (Remember, except B & F)
· This simple principle also makes it easy to notate diminished or augmented fifths instantly without counting steps, by making a perfect fifth by matching the accidentals and then adjusting one of the notes a half step down or up
· This system works just as well for descending intervals as ascending ones
· This system also makes it very easy to notate sixths as well. A M6 interval is a whole step bigger than a P5. To quickly write a M6 above any note, you can easily find the note a P5 through this matching principle, and then go up one more whole step (and letter name) to make a M6. Use the same process for quickly notating a m6 interval, since a m6 is a half step bigger than a P5 – figure out the note that is a P5 away, and then go a half step further. Since this process works just as well when you are notating descending intervals as well as ascending - it might be faster and more accurate than whatever system you are using. Try it!
· Just don’t forget about B & F! This B & F fifth is one half step too small – a d5. So to make it perfect, an accidental will be needed to raise the top note, or lower the bottom one, one half step. In other words, it will NOT “match.”
Here are some examples of perfect and not perfect fifths. In the perfect fifths, notice how the accidentals are either the same on both notes, or there are no accidentals on both notes (both notes are natural.) Notice that on the fifths that are not perfect, the accidentals are NOT the same on both notes - they do not match. One note has a flat and the other doesn't, or one note has a sharp and the other doesn't. Or one might have a sharp and the other a double sharp. These are indications that the fifth is not perfect. When you see that a fifth is not perfect, it is quite easy to tell whether it is diminished or augmented by observing whether the accidental makes the distance between the notes bigger or smaller.

Here is the secret to why this simple principle works: if you make all the fifths on the white keys (shown notated below) you will find that they are all the same size (P5), except the one from B to F. That means when we raise or lower both notes with the same accidental, the notes are both higher or lower by the same amount but the distance between the two notes is still the same.
A perfect fifth is three and one half steps. If we look at all the fifths on the white keys of the piano, it is easy to see the size of each of each.
Let’s first look at the fifth from C to G on the keyboard. You can observe that the distances between the white keys contained within this fifth are mostly whole steps, but notice that there is also one half step enclosed between these notes, E & F. If we count out these whole and half steps, we would find that this P5 contains three and one half steps. This will be our “model P5” – the one we compare all the others to.
If you make all the other fifths on the white keys, such as D to A, E to B, etc., you will see that, just like the fifth from C to G, they too have one half step in between their notes and all the other white keys between are whole steps, except for the fifth on B & F. The fifth from B to F contains two half steps, between B & C and E & F, so it is only three whole steps in size. This means this particular fifth is one half step too short to be a P5. The fifth from B to F is therefore a d5.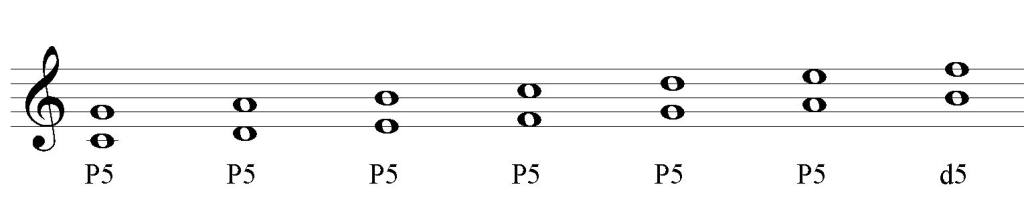
Above is a diagram of all the fifths on the white keys. No matter what octave or clef these fifths occur in, they are always this same size.
When you sharp both notes, you are raising each one a half step, but the distance between them stays the same. As long as you raise or lower both notes by the same amount, with the same kind of accidental (match them up), the fifth will be perfect. Match away!
This principle will greatly speed up your notation of intervals, especially some of the larger ones (4ths and 5ths, as well as help building 6ths).
It can also help you quickly determine the quality of a triad: if you see a diminished fifth in a chord (easy to spot, because the accidentals don’t match in a way that makes it smaller, for example, the top note has a flat but not the bottom one), you likely have a diminished triad. Likewise if you see a fifth that doesn’t match, and it is too big by a half step, you probably have an augmented triad. If the notes of the fifth “match,” then it is a P5 and the triad will be either major or minor. Those two types of triads contain perfect fifths, so you’ll still have to look at the third to tell which kind it is. But in any case, you can check the quality of the fifth instantly, by eye, and have a good idea what kind of triad you are dealing with. NO need to look at a piano, or count out whole and half steps.
Once you get comfortable with this, it is incredibly fast, MUCH more accurate that counting steps, and works both ascending and descending. This matching principle is useful in so many ways.
Perfect fourths work just the same way - all are perfect, except the one from F to B. But in this case, the one from F to B is one half step too large, an A4. With all other fourths, the accidentals can be matched so that they are perfect, but F & B will have to be made smaller by one half step in order to be a P4 – by either lowering the top note, or raising the bottom one one half step.
|
